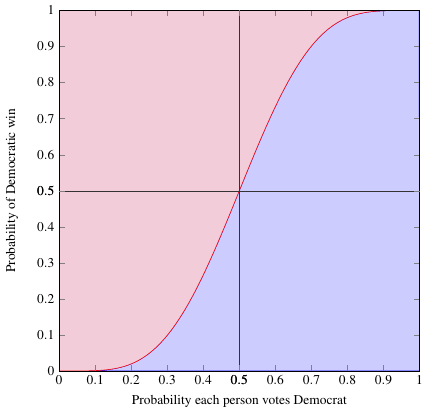
It’s Election Day (in the US), and I have a relevant post I’ve been meaning to do for a while.

Suppose you have a binary experiment, one which has two possible outcomes with probabilities $p$ and $q = 1-p$. For example, voting. (Pretend there are only 2 parties) Overall, let’s say people vote Democrat with probability $p$ and Republican with probability $q$. Now suppose a large number $N$ of people all go out to vote; what can you say about the results?

In a statistical experiment like this, the possible results are drawn from a binomial distribution, in which the probability of getting $n$ Democratic votes (and $N - n$ Republican) is

The probability that the Democrats will come out ahead is just the sum of all the probabilities for all the outcomes where $n$ is more than half of the total vote: we start at $n = \floor*{\frac{N}{2} + 1}$, which is the first integer greater than $\frac{N}{2}$, and add up probabilities all the way to $n = N$.